
How often have you heard or said after looking through a mathematics program, “There’s just not enough rigor”? If you dig deeper into these thoughts, what you will find is that what is truly being referred to is “difficulty.” The issue is that “difficult” is not synonymous with “rigorous,” and more importantly, simply using difficult tasks with students does not lead to them becoming mathematically proficient.
Rigor refers to a balance between conceptual understanding, procedural fluency, and application. The idea is if students understand mathematics conceptually, they will be more likely to apply procedures flexibly and precisely. Rigor is best accomplished by teaching concepts before procedures. The key here is that students should make sense of the mathematics by exploring “the why” before they develop fluency—I guess this could be thought of as “the what.” It is time to acknowledge that this isn’t enough.
An Example From the Math Classroom
How often have you spent considerable time and energy teaching conceptually first before teaching students procedures and then been frustrated with the results? An example of this might be supporting students to make sense of subtracting multidigit numbers using base ten blocks. Why is it that the same students who are successful using manipulatives to subtract with regrouping seem to forget how to regroup later on when using the standard algorithm for multidigit subtraction? It might be that the conceptual understanding the students developed using the base ten blocks was not explicitly linked to the process of using the standard algorithm. Let’s say a class of students is provided with the following word problem:
Alex has 435 pencils. She donates 159 pencils to the school. How many pencils does she have now? Solve the problem using base ten blocks and justify your reasoning.
Students represent 435 pencils with 4 hundred blocks, 3 ten blocks, and 5 ones. The students solve the task and then explain that they removed 1 hundred block to subtract the hundred in 159. They need to exchange another hundred block for 10 ten blocks before they could remove 5 of them for the 50 pencils. Finally, the students exchange one of the remaining 8 ten blocks for 10 ones so they could remove 9 ones, leaving them with 6 ones left. The students share that Alex would have 276 pencils after donating 159 pencils to the school.
The process students used and the way they explained and justified their process provides a good indication that students understand the concept of subtracting with regrouping. They seem ready to learn the algorithm. If a teacher next teaches the standard algorithm for subtracting multidigit numbers with regrouping, the concept clearly preceded the procedure. However, the concept and associated skill may not have been connected. Connecting the steps of the standard algorithm represented symbolically to the process of making exchanges and subtracting with the base ten blocks physically is crucial.
The Learning Arc
When my co-authors and I sat down to create Into Math, we wanted to make the link between concepts and skills explicit. We designed a learning arc (see diagram) that supports students to build understanding of concepts, connect that conceptual understanding to skills, and then apply and practice those skills. This is accomplished with a coherent scope and sequence that is based on three types of lessons:
- Build Understanding
- Connect Concepts and Skills
- Apply and Practice Skills
This is further supported by the experiences offered to students within the lessons. Instruction focused on building students’ conceptual understanding and helping students link that understanding to more efficient processes and skills should begin with students doing the sense making.
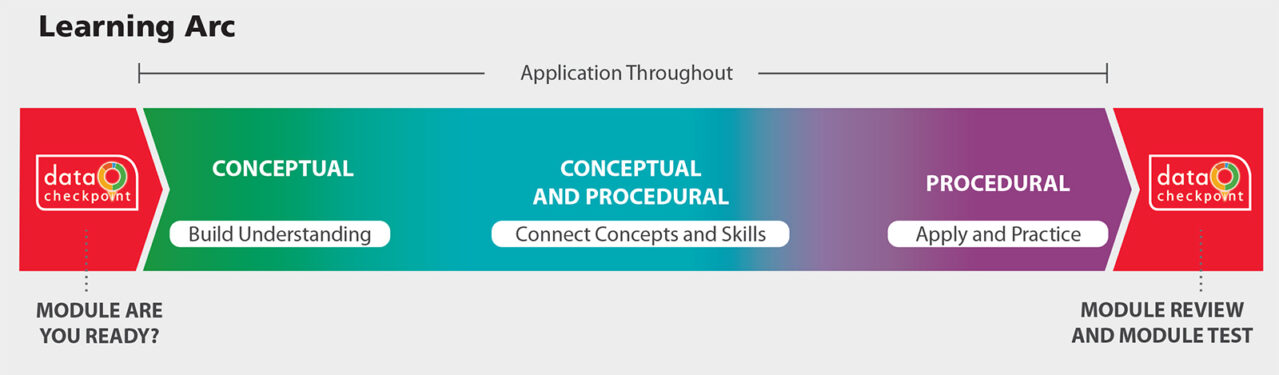
We support students to engage in lessons with these foci by introducing the lessons with a Spark Your Learning task. These tasks are designed so that students can use their own strategies to make sense of the mathematics topic. Students use these experiences to develop productive perseverance. As students move through the learning arc, their informal thinking is developed into conceptual understanding and connected to more efficient procedures. Students go on to apply and practice these new skills with rich mathematical tasks.
Rigor is achieved as students learn from all of these experiences and apply their conceptual understanding to procedural fluency and application. When teachers support students to transition through this learning arc, they see students who are successfully making sense of concepts and applying that understanding as they exhibit procedural fluency.
The views expressed in this article are those of the author and do not necessarily represent those of HMH.
***
Dr. Juli K. Dixon is an author of Into Math. Learn more about the HMH program for K-8 students here.

















